About Efficiency of High-order Harmonic Generation in Attosecond Physics

For the first time, the interaction between Hydrogen atom and Free-Electron Lasers (FEL) is simulated. The conversion efficiency of High-order Harmonic Generation (HHG) can be enhanced by utilizing a two-color free electron laser with frequency multiplication. It is found that the conversion efficiency of HHG is improved to the largest extent when fourth-fold frequency multiplication is introduced into two-color FEL. The microscopic mechanism of improving the efficiency of HHG is analyzed and discussed.

Attosecond physics belongs to one of the areas in ultrafast science since it provides the feasibility to observe the motion of electronic wave packets inside atoms, molecules, and solids [1,2]. Currently, it is established as a mature research field, which offers effective methods for the investigation of fundamental electronic processes [3]. The field of attosecond physics was justly awarded the Nobel Prize for physics in the year 2023.

The appearance of attosecond pulses results from High-order Harmonic Generation (HHG) in gases, which occurs as a strong nonlinear process when an intense and short-light pulse is concentrated onto a gas medium. Subsequently, coherent Extreme Ultraviolet (XUV) radiation with pulse duration down to the attosecond range is obtained in this HHG process [4]. The physical processes causing HHG can be explained utilizing a quasi-classical three-step model [5]. This model is rather useful and interesting, because it gives a clear physical picture regarding the production of very short radiation bursts, with duration in the attosecond regime. Additionally, it has already been confirmed by a previous experiment [6]. Although HHG is believed to have broad applicable prospects, there are certain discrepancies for practical applications of HHG, which is mainly due to the low conversion efficiencies of HHG. Hence, how to improve the efficiency of HHG has become one of the most important topics in the investigations of HHG. In this work, numerical simulations have been conducted to improve the efficiency of HHG. It is demonstrated that the efficiency of HHG can be enhanced by using a two-color Free Electron Laser (FEL).

In this article, a hydrogen atom is chosen to delve into enhancing the efficiency of HHG induced by a two-color free electron laser. FREE-ELECTRON LASERS (FEL) can give rise to coherent light by accelerating a beam of relativistic electrons injected into an undulator magnet [7]. Similar to synchrotron radiation, FEL is a device that is capable of generating coherent radiation due to stimulated bremsstrahlung. Free-electron lasers have many advantages, including useful radiation with wavelengths ranging from 50 nm and 150 nm [8,9] and operating in an ultrafast pulse mode [10,11]. Therefore, FEL will be regarded as an important machine for applications in many subjects, including physics and chemistry [12-14].

We solve the time-dependent Schrödinger equation numerically to investigate the interaction between free electron laser and hydrogen atom. One-dimensional model is adopted because of its simplicity and high efficiency, as well as the convenience for treating problems in atomic physics. The one-dimensional time-dependent Schrödinger equation for the interaction between Free Electron Laser (FEL) and hydrogen atom can be expressed as:

$i\frac{\partial }{\partial t}\Psi \left(x,t\right)=\left[-\frac{1}{2}\frac{{\partial }^2}{\partial x^2}+V_a(x)+E(t)x\right]\Psi (x,t)$

Where V_a (x) is atomic potential, which has the functional form $V_a(x)=-\frac{1}{\sqrt{x^2+2}}.$

E(t) x is the interaction potential between electron and FEL. Ψ (x,t) Denotes the wave function of the investigated system at time t. For the purpose of calculating the HHG subjected to free-electron laser, the form of two-color FEL is set as $E(t)=E_{0}f(t)[\sin \left({\omega }_{0}t\right)+r\sin (n{\omega }_{0}t)]$ , where one-color free electron laser corresponds to r = 0 and two-color free electron laser has r = 0.2. ω₀ and n denote the fundamental frequency and frequency multiplication number, respectively. f(t) refers to pulse envelope [15]. The split-operate method [16] is adopted to execute the wavepacket propagation. Additionally, it is necessary to employ the absorption potential so that the time-dependent wave function can eschew boundary reflections [17].

The harmonic spectrum can be expressed as where a(ω) is the Fourier transformation of the time-dependent acceleration a(t), which is obtained from the time-dependent wavefunction Ψ (x,t),

$a(t)=\langle \Psi \left(x,t\right)|-\frac{dV\left(x\right)}{dx}-E(t)|\Psi \left(x,t\right)\rangle$

The ionization probability I(t) means the total electron population minus the population in certain bound states. $I(t)=1-{\displaystyle \sum _{k=0}^n{|\langle {\varphi }_k\left(x\right)|\Psi \left(x,t\right)\rangle |}^2}$

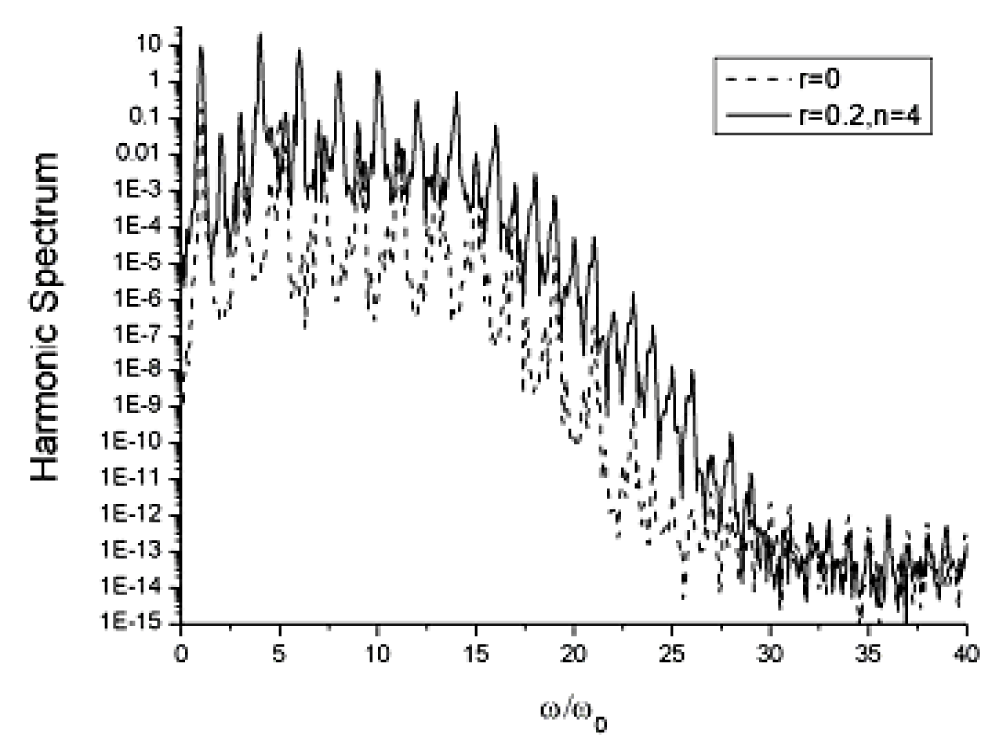
Although there are some experimental quests for better conversion efficiency of HHG [18-20], very little theoretical work has been performed regarding this topic. The emphasis of this work is to test whether the efficiency of HHG can be enhanced by two-color FEL with frequency multiplication. Figure 1 demonstrates the harmonic spectrum under the influence of one-color (r = 0) and two-color (r = 0.2, n = 4) free electron lasers. It is shown that the plateau region is enhanced by 10 to 100 times although the amplitude of frequency-multipled FEL is only one-fifth (r = 0.2), compared with that in one-color FEL. Additionally, even-order harmonics appear, which is attributed to the interference of two-color free electron lasers. These mean that the efficiency of HHG can be enhanced because the paths of electronic transition have increased with the addition of two-color FEL with frequency multiplication.

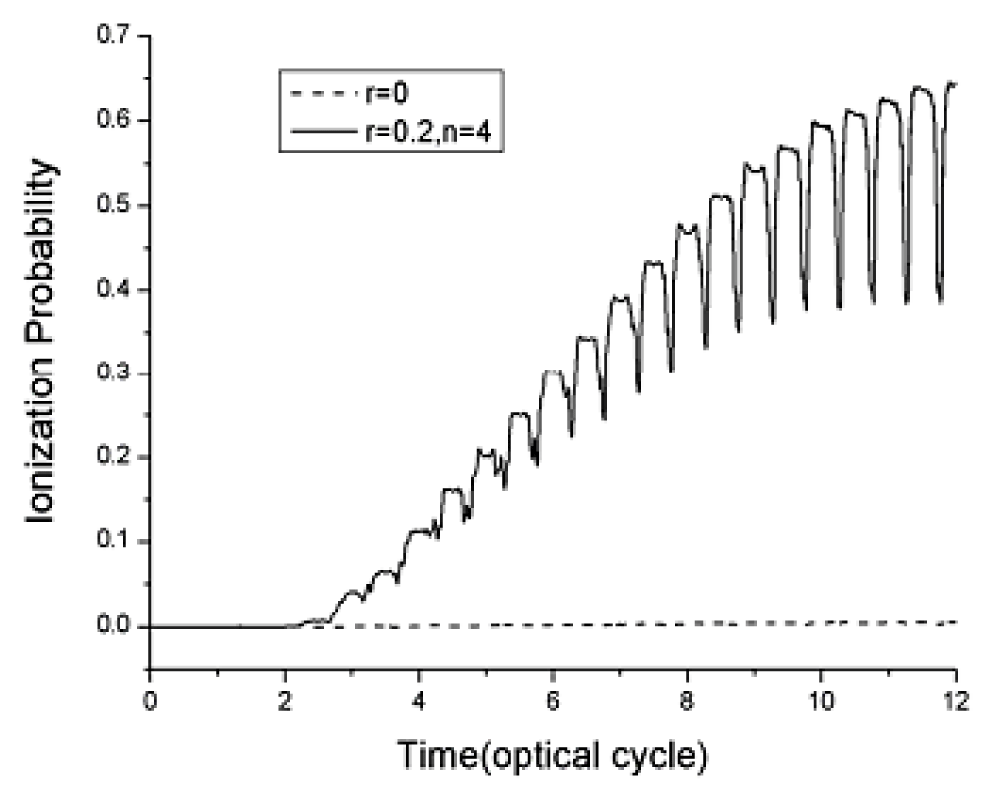
Figure 2 illustrates the ionization probability under the influence of one-color and two-color FELs. It can be seen that the Ionization Probability (IP) caused by two-color FEL is larger than the IP of one-color FEL, which means that the population of continuum states increases. There is more prominent ionization with the addition of frequency-multiple two-color FEL. Subsequently, the number of electrons that return to the ground state and combine with nuclei increases, compared with the number under the radiation of one-color FEL. As a result, the number of photons emitted is enlarged correspondingly. Therefore, the number of HHG photons increases dramatically, which has enhanced the efficiency of HHG greatly.

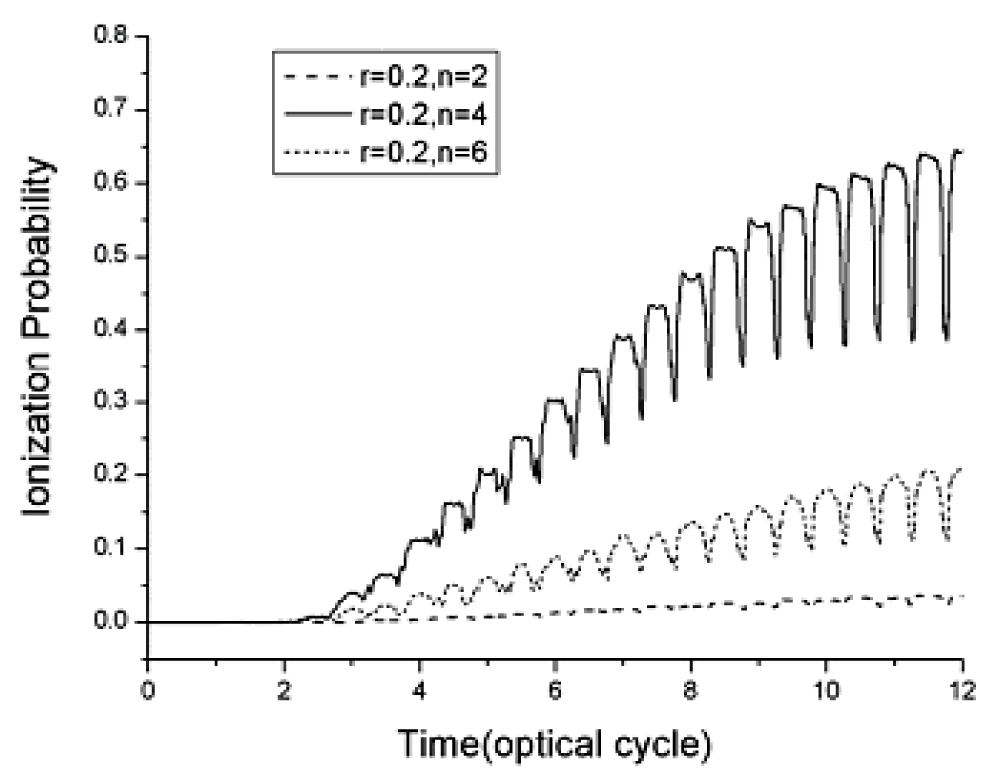
It is necessary to compare the numbers of harmonic, which can impact the efficiency of HHG more or less. Figure 3 exhibits the harmonic spectrum under the radiation of different two-color FELs. It is demonstrated that the efficiency of HHG is enhanced to the most extent with the addition of fourth-fold frequency two-color FEL. Figure 4 exhibits the ionization probability under the radiation of different two-color FELs. It is shown that the ionization probability under the radiation of fourth-fold frequency two-color FEL is much larger than those under the radiation of twice and sixth-fold frequency two-color FELs. Since the energy of the fourth-fold frequency is very adjacent to the ionization energy of the first excited state, which makes the ionization probability of the first excited state quite large after absorbing one photon from the fourth-fold frequency FEL, thus, the population of the first excited state is little with the addition of fourth-fold frequency two-color FEL. Concomitantly, the transition probabilities from the continuum state to the ground state and each excited state are increased, which can help enhance the efficiency of HHG.

To summarize, the ionization probability is increased with the addition of a fourth-fold frequency two-color FEL. Meanwhile, the number of emitted HHG photons rises greatly, which thus enhances the efficiency of HHG. Therefore, we can conclude that the application of two-color FEL with proper frequency multiplication is an effective method of increasing the efficiency of HHG. It should be mentioned that there are some other factors, which can have some impact on improving the efficiency of HHG. For instance, the phase of the frequency-multiple FEL can influence the efficiency of HHG, which deserves further study in the future. Overall, it is expected that more feasible methods will be found to enhance the efficiency of HHG, which can make HHG serve as a benefit for humankind.

Support of this work by the National Natural Science Foundation of China is gratefully acknowledged.

1. Corkum PB, Krausz F. Attosecond science. Nat Phys. 2007;3:381-387. https://ui.adsabs.harvard.edu/abs/2007NatPh...3..381C/abstract
2. Krausz F, Ivanov M. Attosecond physics. Rev Mod Phys. 2009;81:163-234. https://journals.aps.org/rmp/abstract/10.1103/RevModPhys.81.163
3. Calegari F, Ayuso D, Trabattoni A, Belshaw L, De Camillis S, Anumula S, et al. A few-cycle laser pulse at 400 nm driving high-harmonic generation. Science. 2014;346:336-339. https://doi.org/10.1126/science.1254061
4. Krause JL, Kenneth J. Schafer, Kenneth C. Calculation of photoemission from atoms subject to intense laser fields. Phys Rev A. 1992;45:4998. https://journals.aps.org/pra/abstract/10.1103/PhysRevA.45.4998
5. Schafer KJ, Yang B, DiMauro LF, Kulander KC. Above-threshold ionization beyond the high-frequency approximation. Phys Rev Lett. 1993;70:1599. https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.70.1599
6. Paul PM, Toma ES, Breger P, Mullot G, Auge F, Balcou P, et al. Observation of a train of attosecond pulses from high harmonic generation. Science. 2001;292:1689. https://doi.org/10.1126/science.1059413
7. Dattoli G, Doria A, Sabia E, Artioli M. Charged Beam Dynamics, Particle Accelerators and Free-electron Lasers. Bristol: IOP Publishing Ltd; 2017. Online ISBN: 978-0-7503-1239-4; Print ISBN: 978-0-7503-1240-0. https://searchworks.stanford.edu/view/12286375
8. Li C, Wei S, Xuewei D, Du L, Wang Q, Zhang W, et al. Chaoyang Li,et al. Nuclear Instruments and Methods in Physics Research A 2015, 783: 65–67. Nucl Instrum Methods Phys Res A. 2015;783:65-67. https://www.sciencedirect.com/science/article/abs/pii/S016890021500131X
9. Wang G, Zhang W, Wu G, Dai D, Yang X, Feng C, et al. Longitudinal phase space manipulation for ultra-relativistic electron beams. Phys Rev ST Accel Beams. 2015;18:060701. https://journals.aps.org/prab/abstract/10.1103/PhysRevSTAB.18.060701
10. Mirian NS, Fraia MD, Spampinati  S, Sottocorona  F, Allaria E, Badano L, et al. Generation and measurement of intense few-femtosecond superradiant extreme-ultraviolet free-electron laser pulses. Nat Photonics. 2021;15:523-529. https://www.nature.com/articles/s41566-021-00815-w
11. Ding T, Rebholz M, Aufleger L, Hartmann M, Stooß V, Magunia A, et al. Measuring the frequency chirp of extreme-ultraviolet free-electron laser pulses by transient absorption spectroscopy. Nat Commun. 2021;12:643. https://www.nature.com/articles/s41467-020-20846-1
12. Jiang S, Su M, Yang S, Wang C, Huang QR, Li G, et al. Vibrational Signature of Dynamic Coupling of a Strong Hydrogen Bond. J Phys Chem Lett. 2021;12(9):2259-2265. https://pubmed.ncbi.nlm.nih.gov/33636082/
13. Fang L, Osipov T, Murphy B, Tarantelli F, Kukk E, Cryan JP, et al. Multiphoton ionization as a clock to reveal molecular dynamics with intense short x-ray free electron laser pulses. Phys Rev Lett. 2012;109(26):263001. https://pubmed.ncbi.nlm.nih.gov/23368555/  
14. Yase S, Nagaya K, Mizoguchi Y, Yao M, Crossover in the photoionization processes of neon clusters with increasing EUV free-electron-laser intensity. Phys Rev A. 2013;88:043203. https://journals.aps.org/pra/abstract/10.1103/PhysRevA.88.043203
15. Luppi E, Head-Gordon M. Computation of high-harmonic generation spectra of H2 and N2 in intense laser pulses using quantum chemistry methods and time-dependent density functional theory. Mol Phys. 2012;110:909-923. https://doi.org/10.1080/00268976.2012.675448
16. Sathyamurthy N, Mahapatra S. Time-dependent quantum mechanical wave packet dynamics. Phys Chem Chem Phys. 2021;23(13):7586-614. https://pubs.rsc.org/en/content/articlehtml/2021/cp/d0cp03929b
17. Neuhauser D, Baer M; Kouri DJ. The application of optical potentials for reactive scattering: A case study. J Chem Phys. 1990;93:2499. https://pubs.aip.org/aip/jcp/article-abstract/93/4/2499/672909/The-application-of-optical-potentials-for-reactive
18. Brizuela F, Heyl CM, Rudawski P, Kroon D, Rading L, Dahlström JM, et al. Efficient high-order harmonic generation boosted by below-threshold harmonics. Sci Rep. 2013;3:1410. https://www.nature.com/articles/srep01410
19. Yu A. Excitation and ionization of helium atom induced by intense free-electron laser pulses. Res Rev J Mod Phys. 2023;3(1):11-13. https://www.scieniqpublishers.com/wp-content/uploads/2023/10/Excitation-and-ionization-of-helium-atom-induced-by-intense-free-electron-laser-pulses-2023.pdf
20. Farag A, Nause A. Automated, Convenient and Compact Auto-correlation Measurement for an Ultra-fast Laser Pulse. Instrum Exp Tech. 2020;63:547-550. https://link.springer.com/article/10.1134/S0020441220040028